Split Due to Inconclusiveness theorem (Barseghyan-2015)

This is an answer to the question Mechanism of Mosaic Split that states "When a mosaic split is a result of the acceptance of only one theory, it can only be a result of inconclusive theory assessment."

Split Due to Inconclusiveness theorem was formulated by Hakob Barseghyan in 2015.1 It is currently accepted by Scientonomy community as the best available answer to the question.

Scientonomic History

Acceptance Record

Here is the complete acceptance record of this theory:

Community	Accepted From	Acceptance Indicators	Still Accepted	Accepted Until	Rejection Indicators
Scientonomy	1 January 2016	The theorem became de facto accepted by the community at that time together with the whole theory of scientific change.	Yes

Question Answered

Split Due to Inconclusiveness theorem (Barseghyan-2015) is an attempt to answer the following question: What happens to a mosaic when two or more similar theories are considered equally acceptable by a scientific community? Under what conditions does a mosaic split occur? What happens to a mosaic when it is transformed into two or more mosaics?

See Mechanism of Mosaic Split for more details.

Description

Split due to inconclusiveness can occur when two mutually incompatible theories are accepted simultaneously by the same community.

Reasons

Reason: Split Due to Inconclusiveness theorem Deduction

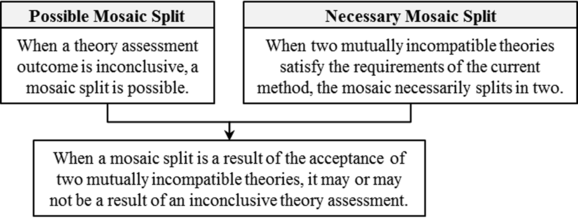
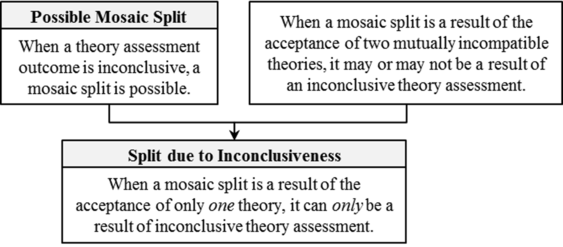
Barseghyan notes that, "when a mosaic split is a result of the acceptance of two new theories, it may or may not be a result of inconclusiveness".1p. 209

"Thus," he concludes, "if we are to detect any instances of inconclusive theory assessment, we must refer to the case of a mosaic split that takes place with only one new theory becoming accepted by one part of the community with the other part sticking to the old theory. This scenario is covered by the possible mosaic split theorem. We can conclude that when a mosaic split takes place with only one new theory involved, this can only indicate that the outcome of the assessment of that theory was inconclusive."1pp. 209-210

This is the deduction of the Split Due to Inconclusiveness Theorem.

This reason for Split Due to Inconclusiveness theorem (Barseghyan-2015) was formulated by Hakob Barseghyan in 2015.1

Edit Delete

If a reason supporting this theory is missing, please add it here.

Questions About This Theory

There are no higher-order questions concerning this theory.

If a question about this theory is missing, please add it here.